
Most races and cultures are known to use a decimal system (base-10) incorporating the numbers zero through ten. Though the elves were also known to use the decimal system, they preferred to use a system of counting in sixes and twelves. This is known as a duodecimal, or base-12 or dozenal, system, and I will try my best to explain how they used this system as simply as possible.
The duodecimal, or base-12 or dozenal, system consists of twelve (12) numbers. It is identical to the decimal (base-10) system of counting for numbers zero through nine, but the numbers 10, 11, and 12 have different notations. In the duodecimal system, 10 = A, 11 = B, and 12 = 10! The numbers 10 and 11 are assigned the letters A and B because they are two-digit numbers. This is so it is understood that 10 and 11 are each complete numbers and not two separate numbers written together (i.e. 1 & 0, 1 & 1).
That leaves how the number 12 = 10. Remember that the duodecimal system is also known as a base-12 system, or dozenal. In this system instead of counting in multiples of ten (10, 100, 100) as in the decimal, base-10 system, you instead count in multiples of 12 giving you 12, 144, 1728. The number 10 in duodecimal means “1 dozen and 0 units,” or 12 & 0 units which equals 12 in the decimal, base-10, system. The number 12 in duodecimal (“1 dozen and 2 units,” or 12 & 2) equals 14 in decimal!
Here is a chart showing the decimal and duodecimal systems as well as the associated elvish tengwa for each number.
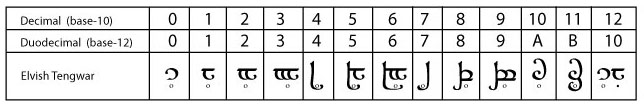
You may have noticed that the tengwar for the number 12 (10 in duodecimal) appears to be backwards, written as 01. That is because it is written backwards. The elves write and read their numbers from right-to-left. Also, to indicate that we are using the duodecimal system, a small circle is drawn under the left-most digit in the number. Small dots, or closed circles, can be drawn under each additional digit in the number.
To better understand this system, let’s write the year 2012 using the duodecimal system and elvish tengwar. First you need to convert the decimal number 2012 to duodecimal. Think only in multiples of 12. Above I mentioned that in the duodecimal system the multiples of 12 were 12, 144, 1728. That is 12¹, 12², and 12³. For this example we should also include one as we need to have a 4-digit significant number. So now we have 12³ 12² 12¹ 1 as the place holders in the number 2012. Determine how many multiples of 12 you need for each digit. For instance, the first digit or place holder signifies 12³ or 1728. 2012 is only divisible by 1728 once, so we have (1×12³) as our first digit. Subtracting 1728 from 2012 we are left with 284 and in the second digit position or 12², which is 144. 284 is only divisible by 144 once, so we now have (1×12²) as our second digit. This leaves us with 140 (by subtracting 144 from 284) and in the third digit position or 12¹. 140 is divisible by 12 eleven (11) times giving us (11×12¹) as our third digit. We are left with 8 for the last digit and since it is a single digit and not divisible by 12 we leave it as it is. I realize this may be confusing, so let me show it to you this way…
2012 = (1×12³) + (1×12²) + (11×12¹) + 8 , remove the plus signs and the powers of 12 you have (1) (1) (11) 8 = 1 1 B 8
Hopefully that will help to clarify how we go from decimal 2012 to duodecimal 11B8.
Now, to write the number using tengwar we first have to write the number backwards, giving us 8B11.
Change each digit to the corresponding tengwa shown in the table above and you have:

Try it yourself with some other numbers, just remember that what would be called tens, hundreds, thousands (10, 100, 1000) in the decimal system of counting are called dozen, gross, great-gross in the duodecimal (12, 144, 1728). I have made a PDF of all the numbers from 0 to 72 in preparation for the elvish calendar I am making, which may help in understanding the duodecimal system. It is available to view/download here. When looking at the list, remember that the tengwar are written in reverse!
This is by far not a complete teaching of how the elves count, I have only focused on the duodecimal system here. More information can be found on Thorsten Renk’s site Parma Tyelpelassiva – The book of silver leaves on the page The Eldarin Numerals.
(The tengwar font used in this post is Tengwar Sindarin.)


0 Comments